Harmonic Series and Bricks

A summation of the form

1/1 + 1/2 + 1/3 + 1/4 + 1/5 ...

Is called the harmonic series. This series diverges. On other words, if you keep adding terms of the series, the sum will grow without a limit. This was proven by Nicole d'Oresme back in the Medieval times. (Believe it or not, people were concerned about this stuff back then.) He grouped the terms of the harmonic series like so:

(1/1) +
(1/2 + 1/3) +
(1/4 + 1/5 + 1/6 + 1/7) +
(1/8 + 1/9 + 1/10 + 1/11 + 1/12 + 1/13 + 1/14 + 1/15) + ...

And noticed that every "chunk" was bigger than the chunks in the following series:

(1/2) +
(1/4 + 1/4) +
(1/8 + 1/8 + 1/8 + 1/8) +
(1/16 + 1/16 + 1/16 + 1/16 + 1/16 + 1/16 + 1/16 + 1/16) + ...

And each of these chunks happen to add up to 1/2.

Therefore, the harmonic series diverges, because it's like adding 1/2 (plus a bit) an infinite number of times.

Now, this fact about the harmonic series produces a counterintuititive reality in the physical world.


A mathematical stack of bricks.

Imagine that you were stacking bricks, and you wanted to create the biggest overhang possible -- you wanted the topmost bricks to jut out sideways. What is the theoretical limit of that overhang? If you analyze the bricks mathematically, the maximum overhang is related to the harmonic series. But the harmonic series has no limit! Conclusion: there is no maximum overhang! You could stack bricks so the top juts out as far as you want.


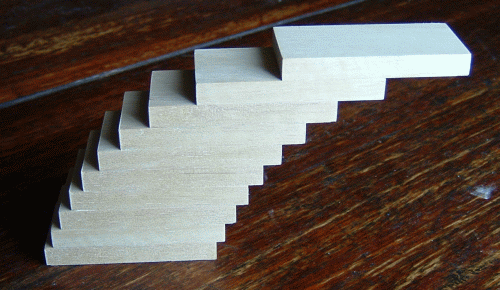
Theory meets reality.

(PDF document with in-depth mathematical discussion here,
and one more picture here. Via Chris Sangwin's Home Page. Also, here's the Mathworld page on the series.)